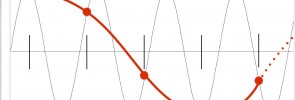
In sampling and quantisation we saw that sampling a signal at a fixed rate means that there is an upper limit on the frequencies that can be represented. This limit is called the Nyquist frequency. Before sampling a signal, we must remove all energy above the Nyquist frequency, and here we will see what would […]
Continue reading...
When we say that a signal is non-stationary we mean that its properties, such as the spectrum, change over time. To analyse signals like this, we need to first assume that these properties do not change over some short period of time, called the frame. We can then analyse individual frames of the signal, one at a […]
Continue reading...
The video and slides from Simon’s keynote are now online under Courses > One-off events.
Continue reading...
A quick introduction to a very simple but widely-applicable model that can perform classification (predicting a discrete label) or regression (predicting a continuous value). The tree is learned from labelled data, using supervised learning. Before watching this video, you might want to check that you understand what Entropy is.
Continue reading...
Time-Domain Pitch Synchronous Overlap and Add (TD-PSOLA) can modify the fundamental frequency and duration of speech signals, without affecting the segment identity – that is, without changing the formants. Normally, it’s an automatic algorithm, but here we do it the hard way – by hand! If you want to follow-along, you will need Audacity and these materials (a […]
Continue reading...
The equation for entropy is very often presented in textbooks without much explanation, other than to say it has the desired properties. Here, I attempt an informal derivation of the equation starting from uniform probability distributions. A good way to think about information is in terms of sending messages. In the video, we send messages […]
Continue reading...
At the Alhambra (Granada, Spain) I saw this nice example of waves from a point source propagating in all directions at a fixed speed.
Continue reading...
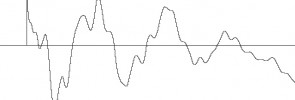
This is the new version. Still under construction.
